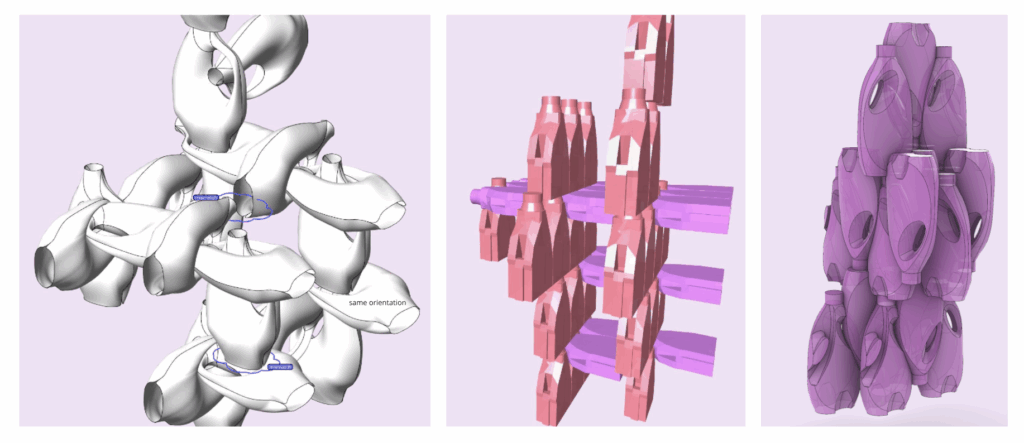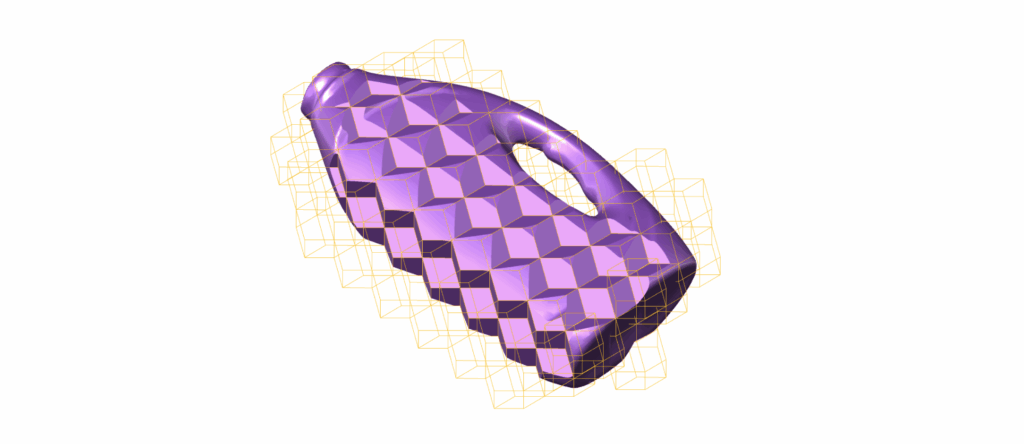
In this part of our ongoing exploration into how detergent bottles can become building blocks, we return to the idea of designing the shape of the bottle. Not by changing the form in complex ways, but by applying what we’ve learned from space-filling geometry.
Instead of completely transforming the bottle, we focused on minimal modifications, while making it suitable for a second life as a modular element.
Learning from the WOBO bottle – (you can read more in our previous blog post) – we were fascinated by how the design embedded reuse directly into the form of the packaging.
We’ve been exploring the idea of giving packaging not just a second life through recycling, but a second function through design. This led us to experiment with how detergent bottles could be transformed into interlocking, modular components, inspired by Japanese joinery, 3D puzzle logic.
WOBO: The Beer Bottle Designed to Build Homes. In the 1960s, Alfred Heineken visited Curaçao and noticed two things: beaches were littered with glass bottles, many from his own brand, and a huge housing shortage. This led him to an idea: what if beer bottles could be used as bricks for housing?
Continue reading
We’ve recreated all five pieces of the Curved Space for 3D printing, transforming them into a toy. The goal was to explore the challenges and problems of the structure on a small scale in order to better understand what needs attention when scaling up.
Continue readingIn an effort to advance discrete element aggregation using the Grasshopper3D plugin WASP (and Andrea building new features into it along the way), we are exploring a ‘sequence-based design’ approach and identified Peter Pearce’s Curved Space Diamond Structure as an ideal foundation.
Using entire bike frames connected at the standard bike joints, 2D lattices can be formed.
Continue reading
Based on the Sommerville tetrahedron, part systems using multiple compatible types can be created that produce more differentiated complex aggregations.
Continue reading
Using an aggregation system based on regular Sommerville-tetra cells, bike frame clusters can be connected along edges.
Continue reading
Combining the concept of bike frames contained in cells with aggregation systems based on the Sommerville-type interconnected structures can be created.
Continue readingAlongside Hill’s tetrahedra there are also other irregular tetrahedral cell types to fill space, of which the Sommerville 1 tetrahedron is a very promising one regarding our intentions.
The particularity here is, that the No. 1 tetrahedron consist of two “brackets” of each 2 triangles with two edges the length of the square root of 3 and one edge length of 2, connected at an right angle at their edges of the length 2.
Continue reading